PHP如何實現繪制二叉樹圖形顯示功能

這篇文章主要介紹PHP如何實現繪制二叉樹圖形顯示功能，文中介紹的非常詳細，具有一定的參考價值，感興趣的小伙伴們一定要看完！

PHP實現繪制二叉樹圖形顯示功能，具體如下：

前言：

最近老師布置了一個作業：理解并實現平衡二叉樹和紅黑樹，本來老師是說用C#寫的，但是我學的C#基本都還給老師了，怎么辦？那就用現在最熟悉的語言PHP來寫吧！

有一個問題來了，書上在講解樹的時候基本上會給出形象的樹形圖。但是當我們自己試著實現某種樹，在調試、輸出的時候確只能以字符的形式順序地輸出。這給調試等方面帶來了很大的不便。然后在各種百度之后，我發現利用PHP實現二叉樹的圖形顯示的資源幾乎是零！好吧，那我就自己個兒實現一個！

效果顯示：

如果我是直接在這一步擺代碼的話，估計大家會比較煩悶，那我就直接上結果吧，后面在補代碼，先激發激發大家的閱讀興趣：

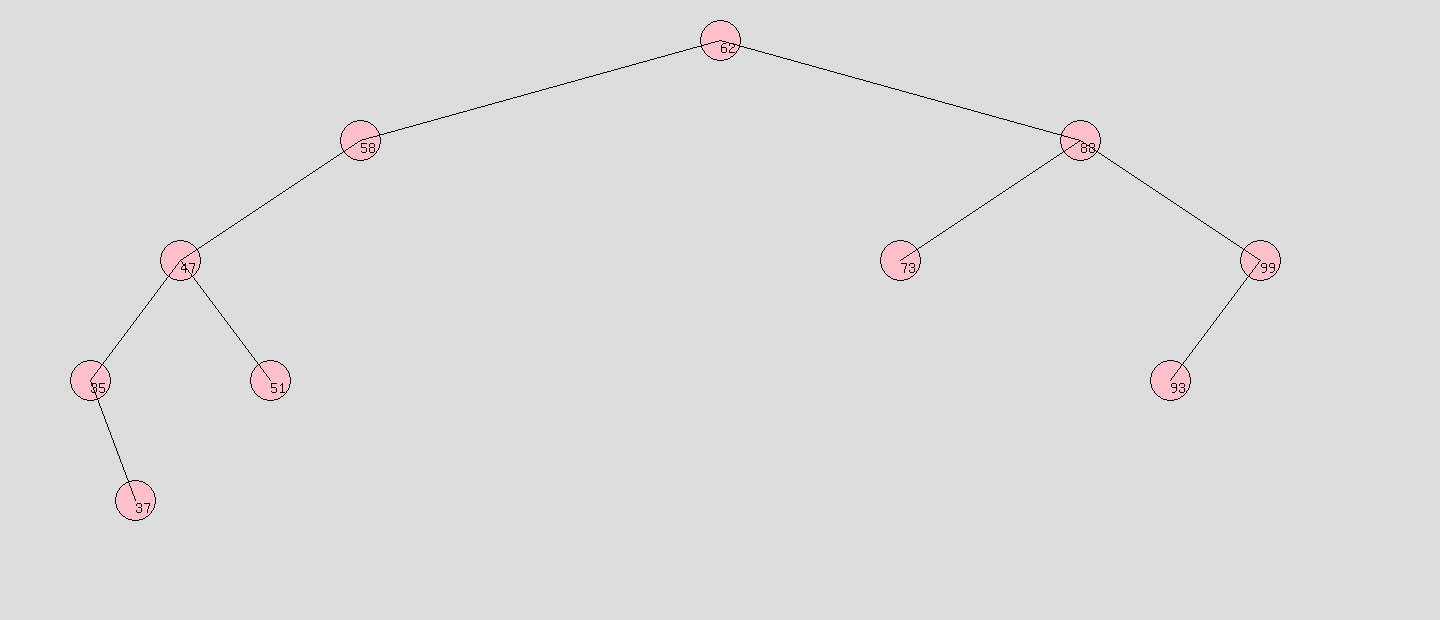
1、搜索二叉樹：

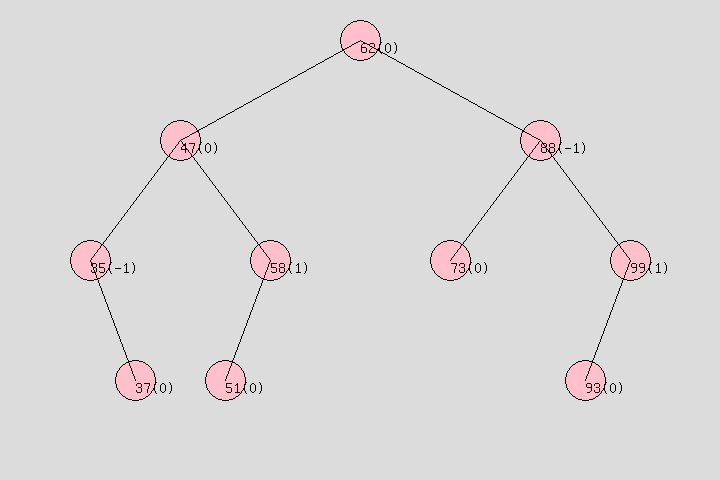
2、平衡二叉樹：

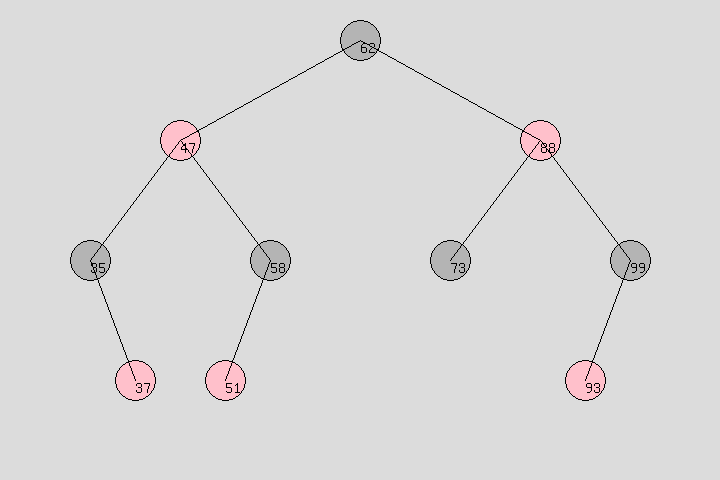
3、紅黑樹：

上代碼：

我們給圖片創建一個類吧，顯得稍微的小高級：

image.php 文件：

<?php
/**
 * author:LSGOZJ
 * description: 繪制二叉樹圖像
 */
class image
{
  //樹相關設置
  //每層之間的間隔高度
  private $level_high = 100;
  //最底層葉子結點之間的寬度
  private $leaf_width = 50;
  //結點圓的半徑
  private $rad = 20;
  //根節點離邊框頂端距離
  private $leave = 20;
  //樹（保存樹對象的引用）
  private $tree;
  //樹的層數
  private $level;
  //完全二叉樹中最底層葉子結點數量（計算圖像寬度時用到，論如何實現圖片大小自適應）
  private $maxCount;
  //圖像相關設置
  //畫布寬度
  private $width;
  //畫布高度
  private $height;
  //畫布背景顏色（RGB）
  private $bg = array(
    220, 220, 220
  );
  //節點顏色（搜索二叉樹和平衡二叉樹時用）
  private $nodeColor = array(
    255, 192, 203
  );
  //圖像句柄
  private $image;
  /**
   * 構造函數，類屬性初始化
   * @param $tree 傳遞一個樹的對象
   * @return null
   */
  public function __construct($tree)
  {
    $this->tree = $tree;
    $this->level = $this->getLevel();
    $this->maxCount = $this->GetMaxCount($this->level);
    $this->width = ($this->rad * 2 * $this->maxCount) + $this->maxCount * $this->leaf_width;
    $this->height = $this->level * ($this->rad * 2) + $this->level_high * ($this->level - 1) + $this->leave;
    //1.創建畫布
    $this->image = imagecreatetruecolor($this->width, $this->height); //新建一個真彩色圖像，默認背景是黑色
    //填充背景色
    $bgcolor = imagecolorallocate($this->image, $this->bg[0], $this->bg[1], $this->bg[2]);
    imagefill($this->image, 0, 0, $bgcolor);
  }
  /**
   * 返回傳進來的樹對象對應的完全二叉樹中最底層葉子結點數量
   * @param $level 樹的層數
   * @return 結點數量
   */
  function GetMaxCount($level)
  {
    return pow(2, $level - 1);
  }
  /**
   * 獲取樹對象的層數
   * @param null
   * @return 樹的層數
   */
  function getLevel()
  {
    return $this->tree->Depth();
  }
  /**
   * 顯示二叉樹圖像
   * @param null
   * @return null
   */
  public function show()
  {
    $this->draw($this->tree->root, 1, 0, 0);
    header("Content-type:image/png");
    imagepng($this->image);
    imagedestroy($this->image);
  }
  /**
   * （遞歸）畫出二叉樹的樹狀結構
   * @param $root，根節點（樹或子樹） $i，該根節點所處的層 $p_x，父節點的x坐標 $p_y,父節點的y坐標
   * @return null
   */
  private function draw($root, $i, $p_x, $p_y)
  {
    if ($i <= $this->level) {
      //當前節點的y坐標
      $root_y = $i * $this->rad + ($i - 1) * $this->level_high;
      //當前節點的x坐標
      if (!is_null($parent = $root->parent)) {
        if ($root == $parent->left) {
          $root_x = $p_x - $this->width / (pow(2, $i));
        } else {
          $root_x = $p_x + $this->width / (pow(2, $i));
        }
      } else {
        //根節點
        $root_x = (1 / 2) * $this->width;
        $root_y += $this->leave;
      }
      //畫結點（確定所畫節點的類型（平衡、紅黑、排序）和方法）
      $method = 'draw' . get_class($this->tree) . 'Node';
      $this->$method($root_x, $root_y, $root);
      //將當前節點和父節點連線（黑色線）
      $black = imagecolorallocate($this->image, 0, 0, 0);
      if (!is_null($parent = $root->parent)) {
        imageline($this->image, $p_x, $p_y, $root_x, $root_y, $black);
      }
      //畫左子節點
      if (!is_null($root->left)) {
        $this->draw($root->left, $i + 1, $root_x, $root_y);
      }
      //畫右子節點
      if (!is_null($root->right)) {
        $this->draw($root->right, $i + 1, $root_x, $root_y);
      }
    }
  }
  /**
   * 畫搜索二叉樹結點
   * @param $x，當前節點的x坐標 $y，當前節點的y坐標 $node，當前節點的引用
   * @return null
   */
  private function drawBstNode($x, $y, $node)
  {
    //節點圓的線顏色
    $black = imagecolorallocate($this->image, 0, 0, 0);
    $nodeColor = imagecolorallocate($this->image, $this->nodeColor[0], $this->nodeColor[1], $this->nodeColor[2]);
    //畫節點圓
    imageellipse($this->image, $x, $y, $this->rad * 2, $this->rad * 2, $black);
    //節點圓顏色填充
    imagefill($this->image, $x, $y, $nodeColor);
    //節點對應的數字
    imagestring($this->image, 4, $x, $y, $node->key, $black);
  }
  /**
   * 畫平衡二叉樹結點
   * @param $x，當前節點的x坐標 $y，當前節點的y坐標 $node，當前節點的引用
   * @return null
   */
  private function drawAvlNode($x, $y, $node)
  {
    $black = imagecolorallocate($this->image, 0, 0, 0);
    $nodeColor = imagecolorallocate($this->image, $this->nodeColor[0], $this->nodeColor[1], $this->nodeColor[2]);
    imageellipse($this->image, $x, $y, $this->rad * 2, $this->rad * 2, $black);
    imagefill($this->image, $x, $y, $nodeColor);
    imagestring($this->image, 4, $x, $y, $node->key . '(' . $node->bf . ')', $black);
  }
  /**
   * 畫紅黑樹結點
   * @param $x，當前節點的x坐標 $y，當前節點的y坐標 $node，當前節點的引用
   * @return null
   */
  private function drawRbtNode($x, $y, $node)
  {
    $black = imagecolorallocate($this->image, 0, 0, 0);
    $gray = imagecolorallocate($this->image, 180, 180, 180);
    $pink = imagecolorallocate($this->image, 255, 192, 203);
    imageellipse($this->image, $x, $y, $this->rad * 2, $this->rad * 2, $black);
    if ($node->IsRed == TRUE) {
      imagefill($this->image, $x, $y, $pink);
    } else {
      imagefill($this->image, $x, $y, $gray);
    }
    imagestring($this->image, 4, $x, $y, $node->key, $black);
  }
}

好，現在我們來看看在客戶端如何調用：

client.php

class Client
{
  public static function Main()
  {
    try {
      //實現文件的自動加載
      function autoload($class)
      {
        include strtolower($class) . '.php';
      }
      spl_autoload_register('autoload');
      $arr = array(62, 88, 58, 47, 35, 73, 51, 99, 37, 93);
//      $tree = new Bst();  //搜索二叉樹
      $tree = new Avl();  //平衡二叉樹
//      $tree = new Rbt();  //紅黑樹
      $tree->init($arr);   //樹的初始化
//      $tree->Delete(62);
//      $tree->Insert(100);
//      $tree->MidOrder();  //樹的中序遍歷（這也是調試的一個手段，看看數字是否從小到大排序）
      $image = new image($tree);
      $image->show();  //顯示圖像
    } catch (Exception $e) {
      echo $e->getMessage();
    }
  }
}
Client::Main();

這里用到的那三個樹的類如下：

二叉搜索樹bst.php：

<?php
 /**
 * author:zhongjin
 * description: 二叉查找樹
 */
//結點
class Node
{
  public $key;
  public $parent;
  public $left;
  public $right;
  public function __construct($key)
  {
    $this->key = $key;
    $this->parent = NULL;
    $this->left = NULL;
    $this->right = NULL;
  }
}
//二叉搜索樹
class Bst
{
  public $root;
  /**
   * 初始化樹結構
   * @param $arr 初始化樹結構的數組
   * @return null
   */
  public function init($arr)
  {
    $this->root = new Node($arr[0]);
    for ($i = 1; $i < count($arr); $i++) {
      $this->Insert($arr[$i]);
    }
  }
  /**
   * （對內）中序遍歷
   * @param $root （樹或子樹的）根節點
   * @return null
   */
  private function mid_order($root)
  {
    if ($root != NULL) {
      $this->mid_order($root->left);
      echo $root->key . " ";
      $this->mid_order($root->right);
    }
  }
  /**
   * （對外）中序遍歷
   * @param null
   * @return null
   */
  public function MidOrder()
  {
    $this->mid_order($this->root);
  }
  /**
   * 查找樹中是否存在$key對應的節點
   * @param $key 待搜索數字
   * @return $key對應的節點
   */
  function search($key)
  {
    $current = $this->root;
    while ($current != NULL) {
      if ($current->key == $key) {
        return $current;
      } elseif ($current->key > $key) {
        $current = $current->left;
      } else {
        $current = $current->right;
      }
    }
    return $current;
  }
  /**
   * 查找樹中的最小關鍵字
   * @param $root 根節點
   * @return 最小關鍵字對應的節點
   */
  function search_min($root)
  {
    $current = $root;
    while ($current->left != NULL) {
      $current = $current->left;
    }
    return $current;
  }
  /**
   * 查找樹中的最大關鍵字
   * @param $root 根節點
   * @return 最大關鍵字對應的節點
   */
  function search_max($root)
  {
    $current = $root;
    while ($current->right != NULL) {
      $current = $current->right;
    }
    return $current;
  }
  /**
   * 查找某個$key在中序遍歷時的直接前驅節點
   * @param $x 待查找前驅節點的節點引用
   * @return 前驅節點引用
   */
  function predecessor($x)
  {
    //左子節點存在，直接返回左子節點的最右子節點
    if ($x->left != NULL) {
      return $this->search_max($x->left);
    }
    //否則查找其父節點，直到當前結點位于父節點的右邊
    $p = $x->parent;
    //如果x是p的左孩子，說明p是x的后繼，我們需要找的是p是x的前驅
    while ($p != NULL && $x == $p->left) {
      $x = $p;
      $p = $p->parent;
    }
    return $p;
  }
  /**
   * 查找某個$key在中序遍歷時的直接后繼節點
   * @param $x 待查找后繼節點的節點引用
   * @return 后繼節點引用
   */
  function successor($x)
  {
    if ($x->right != NULL) {
      return $this->search_min($x->right);
    }
    $p = $x->parent;
    while ($p != NULL && $x == $p->right) {
      $x = $p;
      $p = $p->parent;
    }
    return $p;
  }
  /**
   * 將$key插入樹中
   * @param $key 待插入樹的數字
   * @return null
   */
  function Insert($key)
  {
    if (!is_null($this->search($key))) {
      throw new Exception('結點' . $key . '已存在，不可插入！');
    }
    $root = $this->root;
    $inode = new Node($key);
    $current = $root;
    $prenode = NULL;
    //為$inode找到合適的插入位置
    while ($current != NULL) {
      $prenode = $current;
      if ($current->key > $inode->key) {
        $current = $current->left;
      } else {
        $current = $current->right;
      }
    }
    $inode->parent = $prenode;
    //如果$prenode == NULL， 則證明樹是空樹
    if ($prenode == NULL) {
      $this->root = $inode;
    } else {
      if ($inode->key < $prenode->key) {
        $prenode->left = $inode;
      } else {
        $prenode->right = $inode;
      }
    }
    //return $root;
  }
  /**
   * 在樹中刪除$key對應的節點
   * @param $key 待刪除節點的數字
   * @return null
   */
  function Delete($key)
  {
    if (is_null($this->search($key))) {
      throw new Exception('結點' . $key . "不存在，刪除失敗！");
    }
    $root = $this->root;
    $dnode = $this->search($key);
    if ($dnode->left == NULL || $dnode->right == NULL) { #如果待刪除結點無子節點或只有一個子節點，則c = dnode
      $c = $dnode;
    } else { #如果待刪除結點有兩個子節點，c置為dnode的直接后繼，以待最后將待刪除結點的值換為其后繼的值
      $c = $this->successor($dnode);
    }
    //無論前面情況如何，到最后c只剩下一邊子結點
    if ($c->left != NULL) {
      $s = $c->left;
    } else {
      $s = $c->right;
    }
    if ($s != NULL) { #將c的子節點的父母結點置為c的父母結點，此處c只可能有1個子節點，因為如果c有兩個子節點，則c不可能是dnode的直接后繼
      $s->parent = $c->parent;
    }
    if ($c->parent == NULL) { #如果c的父母為空，說明c=dnode是根節點，刪除根節點后直接將根節點置為根節點的子節點，此處dnode是根節點，且擁有兩個子節點，則c是dnode的后繼結點，c的父母就不會為空，就不會進入這個if
      $this->root = $s;
    } else if ($c == $c->parent->left) { #如果c是其父節點的左右子節點，則將c父母的左右子節點置為c的左右子節點
      $c->parent->left = $s;
    } else {
      $c->parent->right = $s;
    }
    #如果c!=dnode，說明c是dnode的后繼結點，交換c和dnode的key值
    if ($c != $dnode) {
      $dnode->key = $c->key;
    }
    #返回根節點
//    return $root;
  }
  /**
   * （對內）獲取樹的深度
   * @param $root 根節點
   * @return 樹的深度
   */
  private function getdepth($root)
  {
    if ($root == NULL) {
      return 0;
    }
    $dl = $this->getdepth($root->left);
    $dr = $this->getdepth($root->right);
    return ($dl > $dr ? $dl : $dr) + 1;
  }
  /**
   * （對外）獲取樹的深度
   * @param null
   * @return null
   */
  public function Depth()
  {
    return $this->getdepth($this->root);
  }
}
?>

平衡二叉樹avl.php：

<?php
 /**
 * author:zhongjin
 * description: 平衡二叉樹
 */
//結點
class Node
{
  public $key;
  public $parent;
  public $left;
  public $right;
  public $bf; //平衡因子
  public function __construct($key)
  {
    $this->key = $key;
    $this->parent = NULL;
    $this->left = NULL;
    $this->right = NULL;
    $this->bf = 0;
  }
}
//平衡二叉樹
class Avl
{
  public $root;
  const LH = +1; //左高
  const EH = 0;  //等高
  const RH = -1; //右高
  /**
   * 初始化樹結構
   * @param $arr 初始化樹結構的數組
   * @return null
   */
  public function init($arr)
  {
    $this->root = new Node($arr[0]);
    for ($i = 1; $i < count($arr); $i++) {
      $this->Insert($arr[$i]);
    }
  }
  /**
   * （對內）中序遍歷
   * @param $root （樹或子樹的）根節點
   * @return null
   */
  private function mid_order($root)
  {
    if ($root != NULL) {
      $this->mid_order($root->left);
      echo $root->key . "-" . $root->bf . " ";
      $this->mid_order($root->right);
    }
  }
  /**
   * （對外）中序遍歷
   * @param null
   * @return null
   */
  public function MidOrder()
  {
    $this->mid_order($this->root);
  }
  /**
   * 將以$root為根節點的最小不平衡二叉樹做右旋處理
   * @param $root（樹或子樹）根節點
   * @return null
   */
  private function R_Rotate($root)
  {
    $L = $root->left;
    if (!is_NULL($root->parent)) {
      $P = $root->parent;
      if ($root == $P->left) {
        $P->left = $L;
      } else {
        $P->right = $L;
      }
      $L->parent = $P;
    } else {
      $L->parent = NULL;
    }
    $root->parent = $L;
    $root->left = $L->right;
    $L->right = $root;
    //這句必須啊！
    if ($L->parent == NULL) {
      $this->root = $L;
    }
  }
  /**
   * 將以$root為根節點的最小不平衡二叉樹做左旋處理
   * @param $root（樹或子樹）根節點
   * @return null
   */
  private function L_Rotate($root)
  {
    $R = $root->right;
    if (!is_NULL($root->parent)) {
      $P = $root->parent;
      if ($root == $P->left) {
        $P->left = $R;
      } else {
        $P->right = $R;
      }
      $R->parent = $P;
    } else {
      $R->parent = NULL;
    }
    $root->parent = $R;
    $root->right = $R->left;
    $R->left = $root;
    //這句必須啊！
    if ($R->parent == NULL) {
      $this->root = $R;
    }
  }
  /**
   * 對以$root所指結點為根節點的二叉樹作左平衡處理
   * @param $root（樹或子樹）根節點
   * @return null
   */
  public function LeftBalance($root)
  {
    $L = $root->left;
    $L_bf = $L->bf;
    switch ($L_bf) {
      //檢查root的左子樹的平衡度，并作相應的平衡處理
      case self::LH:  //新結點插入在root的左孩子的左子樹上，要做單右旋處理
        $root->bf = $L->bf = self::EH;
        $this->R_Rotate($root);
        break;
      case self::RH:  //新節點插入在root的左孩子的右子樹上，要做雙旋處理
        $L_r = $L->right;  //root左孩子的右子樹根
        $L_r_bf = $L_r->bf;
        //修改root及其左孩子的平衡因子
        switch ($L_r_bf) {
          case self::LH:
            $root->bf = self::RH;
            $L->bf = self::EH;
            break;
          case self::EH:
            $root->bf = $L->bf = self::EH;
            break;
          case self::RH:
            $root->bf = self::EH;
            $L->bf = self::LH;
            break;
        }
        $L_r->bf = self::EH;
        //對root的左子樹作左平衡處理
        $this->L_Rotate($L);
        //對root作右平衡處理
        $this->R_Rotate($root);
    }
  }
  /**
   * 對以$root所指結點為根節點的二叉樹作右平衡處理
   * @param $root（樹或子樹）根節點
   * @return null
   */
  public function RightBalance($root)
  {
    $R = $root->right;
    $R_bf = $R->bf;
    switch ($R_bf) {
      //檢查root的右子樹的平衡度，并作相應的平衡處理
      case self::RH:  //新結點插入在root的右孩子的右子樹上，要做單左旋處理
        $root->bf = $R->bf = self::EH;
        $this->L_Rotate($root);
        break;
      case self::LH:  //新節點插入在root的右孩子的左子樹上，要做雙旋處理
        $R_l = $R->left;  //root右孩子的左子樹根
        $R_l_bf = $R_l->bf;
        //修改root及其右孩子的平衡因子
        switch ($R_l_bf) {
          case self::RH:
            $root->bf = self::LH;
            $R->bf = self::EH;
            break;
          case self::EH:
            $root->bf = $R->bf = self::EH;
            break;
          case self::LH:
            $root->bf = self::EH;
            $R->bf = self::RH;
            break;
        }
        $R_l->bf = self::EH;
        //對root的右子樹作右平衡處理
        $this->R_Rotate($R);
        //對root作左平衡處理
        $this->L_Rotate($root);
    }
  }
  /**
   * 查找樹中是否存在$key對應的節點
   * @param $key 待搜索數字
   * @return $key對應的節點
   */
  public function search($key)
  {
    $current = $this->root;
    while ($current != NULL) {
      if ($current->key == $key) {
        return $current;
      } elseif ($current->key > $key) {
        $current = $current->left;
      } else {
        $current = $current->right;
      }
    }
    return $current;
  }
  /**
   * 查找樹中的最小關鍵字
   * @param $root 根節點
   * @return 最小關鍵字對應的節點
   */
  function search_min($root)
  {
    $current = $root;
    while ($current->left != NULL) {
      $current = $current->left;
    }
    return $current;
  }
  /**
   * 查找樹中的最大關鍵字
   * @param $root 根節點
   * @return 最大關鍵字對應的節點
   */
  function search_max($root)
  {
    $current = $root;
    while ($current->right != NULL) {
      $current = $current->right;
    }
    return $current;
  }
  /**
   * 查找某個$key在中序遍歷時的直接前驅節點
   * @param $x 待查找前驅節點的節點引用
   * @return 前驅節點引用
   */
  private function predecessor($x)
  {
    //左子節點存在，直接返回左子節點的最右子節點
    if ($x->left != NULL) {
      return $this->search_max($x->left);
    }
    //否則查找其父節點，直到當前結點位于父節點的右邊
    $p = $x->parent;
    //如果x是p的左孩子，說明p是x的后繼，我們需要找的是p是x的前驅
    while ($p != NULL && $x == $p->left) {
      $x = $p;
      $p = $p->parent;
    }
    return $p;
  }
  /**
   * 查找某個$key在中序遍歷時的直接后繼節點
   * @param $x 待查找后繼節點的節點引用
   * @return 后繼節點引用
   */
  private function successor($x)
  {
    if ($x->left != NULL) {
      return $this->search_min($x->right);
    }
    $p = $x->parent;
    while ($p != NULL && $x == $p->right) {
      $x = $p;
      $p = $p->parent;
    }
    return $p;
  }
  /**
   * （對內）插入結點，如果結點不存在則插入，失去平衡要做平衡處理
   * @param $root 根節點 $key 待插入樹的數字
   * @return null
   */
  private function insert_node(&$root, $key)
  {
    //找到了插入的位置，插入新節點
    if (is_null($root)) {
      $root = new Node($key);
      //插入結點成功
      return TRUE;
    } else {
      //在樹中已經存在和$key相等的結點
      if ($key == $root->key) {
        //插入節點失敗
        return FALSE;
      } //在root的左子樹中繼續搜索
      elseif ($key < $root->key) {
        //插入左子樹失敗
        if (!($this->insert_node($root->left, $key))) {
          //樹未長高
          return FALSE;
        }
        //成功插入,修改平衡因子
        if (is_null($root->left->parent)) {
          $root->left->parent = $root;
        }
        switch ($root->bf) {
          //原來左右子樹等高，現在左子樹增高而樹增高
          case self::EH:
            $root->bf = self::LH;
            //樹長高
            return TRUE;
            break;
          //原來左子樹比右子樹高，需要做左平衡處理
          case self::LH:
            $this->LeftBalance($root);
            //平衡后，樹并未長高
            return FALSE;
            break;
          //原來右子樹比左子樹高，現在左右子樹等高
          case self::RH:
            $root->bf = self::EH;
            //樹并未長高
            return FALSE;
            break;
        }
      } //在root的右子樹中繼續搜索
      else {
        //插入右子樹失敗
        if (!$this->insert_node($root->right, $key)) {
          //樹未長高
          return FALSE;
        }
        //成功插入,修改平衡因子
        if (is_null($root->right->parent)) {
          $root->right->parent = $root;
        }
        switch ($root->bf) {
          //原來左右子樹等高，現在右子樹增高而樹增高
          case self::EH:
            $root->bf = self::RH;
            //樹長高
            return TRUE;
            break;
          //原來左子樹比右子樹高，現在左右子樹等高
          case self::LH:
            $root->bf = self::EH;
            return FALSE;
            break;
          //原來右子樹比左子樹高，要做右平衡處理
          case self::RH:
            $this->RightBalance($root);
            //樹并未長高
            return FALSE;
            break;
        }
      }
    }
  }
  /**
   * （對外）將$key插入樹中
   * @param $key 待插入樹的數字
   * @return null
   */
  public function Insert($key)
  {
    $this->insert_node($this->root, $key);
  }
  /**
   * 獲取待刪除的節點（刪除的最終節點）
   * @param $key 待刪除的數字
   * @return 最終被刪除的節點
   */
  private function get_del_node($key)
  {
    $dnode = $this->search($key);
    if ($dnode == NULL) {
      throw new Exception("結點不存在！");
      return;
    }
    if ($dnode->left == NULL || $dnode->right == NULL) { #如果待刪除結點無子節點或只有一個子節點，則c = dnode
      $c = $dnode;
    } else { #如果待刪除結點有兩個子節點，c置為dnode的直接后繼，以待最后將待刪除結點的值換為其后繼的值
      $c = $this->successor($dnode);
    }
    $dnode->key = $c->key;
    return $c;
  }
  /**
   * （對內）刪除指定節點，處理該結點往上結點的平衡因子
   * @param $node 最終該被刪除的節點
   * @return null
   */
  private function del_node($node)
  {
    if ($node == $this->root) {
      $this->root = NULL;
      return;
    }
    $current = $node;
    //現在的node只有兩種情況，要么只有一個子節點，要么沒有子節點
    $P = $current->parent;
    //刪除一個結點，第一個父節點的平衡都肯定會發生變化
    $lower = TRUE;
    while ($lower == TRUE && !is_null($P)) {
      //待刪除結點是左節點
      if ($current == $P->left) {
        if($current == $node){
          if (!is_null($current->left)) {
            $P->left = $current->left;
          } else {
            $P->left = $current->left;
          }
        }
        $P_bf = $P->bf;
        switch ($P_bf) {
          case self::LH:
            $P->bf = self::EH;
            $lower = TRUE;
            $current = $P;
            $P = $current->parent;
            break;
          case self::EH:
            $P->bf = self::RH;
            $lower = FALSE;
            break;
          case self::RH:
            $this->RightBalance($P);
            $lower = TRUE;
            $current = $P->parent;
            $P = $current->parent;
            break;
        }
      } //右結點
      else {
        if($current == $node){
          if (!is_null($current->left)) {
            $P->right = $current->left;
          } else {
            $P->right = $current->left;
          }
        }
        $P_bf = $P->bf;
        switch ($P_bf) {
          case self::LH:
            $this->LeftBalance($P);
            $lower = TRUE;
            $current = $P->parent;
            $P = $current->parent;
            break;
          case self::EH:
            $P->bf = self::LH;
            $lower = FALSE;
            break;
          case self::RH:
            $P->bf = self::LH;
            $lower = TRUE;
            $current = $P;
            $P = $current->parent;
            break;
        }
      }
    }
  }
  /**
   * （對外）刪除指定節點
   * @param $key 刪除節點的key值
   * @return null
   */
  public function Delete($key)
  {
    $del_node = $this->get_del_node($key);
    $this->del_node($del_node);
  }
  /**
   * （對內）獲取樹的深度
   * @param $root 根節點
   * @return 樹的深度
   */
  private function getdepth($root)
  {
    if ($root == NULL) {
      return 0;
    }
    $dl = $this->getdepth($root->left);
    $dr = $this->getdepth($root->right);
    return ($dl > $dr ? $dl : $dr) + 1;
  }
  /**
   * （對外）獲取樹的深度
   * @param null
   * @return null
   */
  public function Depth()
  {
    return $this->getdepth($this->root);
  }
}
?>

紅黑樹rbt.php：

<?php
 /**
 * author:zhongjin
 * description: 紅黑樹
 */
//結點
class Node
{
  public $key;
  public $parent;
  public $left;
  public $right;
  public $IsRed; //分辨紅節點或黑節點
  public function __construct($key, $IsRed = TRUE)
  {
    $this->key = $key;
    $this->parent = NULL;
    $this->left = NULL;
    $this->right = NULL;
    //插入結點默認是紅色
    $this->IsRed = $IsRed;
  }
}
//紅黑樹
class Rbt
{
  public $root;
  /**
   * 初始化樹結構
   * @param $arr 初始化樹結構的數組
   * @return null
   */
  public function init($arr)
  {
    //根節點必須是黑色
    $this->root = new Node($arr[0], FALSE);
    for ($i = 1; $i < count($arr); $i++) {
      $this->Insert($arr[$i]);
    }
  }
  /**
   * （對內）中序遍歷
   * @param $root （樹或子樹的）根節點
   * @return null
   */
  private function mid_order($root)
  {
    if ($root != NULL) {
      $this->mid_order($root->left);
      echo $root->key . "-" . ($root->IsRed ? 'r' : 'b') . ' ';
      $this->mid_order($root->right);
    }
  }
  /**
   * （對外）中序遍歷
   * @param null
   * @return null
   */
  public function MidOrder()
  {
    $this->mid_order($this->root);
  }
  /**
   * 查找樹中是否存在$key對應的節點
   * @param $key 待搜索數字
   * @return $key對應的節點
   */
  function search($key)
  {
    $current = $this->root;
    while ($current != NULL) {
      if ($current->key == $key) {
        return $current;
      } elseif ($current->key > $key) {
        $current = $current->left;
      } else {
        $current = $current->right;
      }
    }
    //結點不存在
    return $current;
  }
  /**
   * 將以$root為根節點的最小不平衡二叉樹做右旋處理
   * @param $root（樹或子樹）根節點
   * @return null
   */
  private function R_Rotate($root)
  {
    $L = $root->left;
    if (!is_null($root->parent)) {
      $P = $root->parent;
      if($root == $P->left){
        $P->left = $L;
      }else{
        $P->right = $L;
      }
      $L->parent = $P;
    } else {
      $L->parent = NULL;
    }
    $root->parent = $L;
    $root->left = $L->right;
    $L->right = $root;
    //這句必須啊！
    if ($L->parent == NULL) {
      $this->root = $L;
    }
  }
  /**
   * 將以$root為根節點的最小不平衡二叉樹做左旋處理
   * @param $root（樹或子樹）根節點
   * @return null
   */
  private function L_Rotate($root)
  {
    $R = $root->right;
    if (!is_null($root->parent)) {
      $P = $root->parent;
      if($root == $P->right){
        $P->right = $R;
      }else{
        $P->left = $R;
      }
      $R->parent = $P;
    } else {
      $R->parent = NULL;
    }
    $root->parent = $R;
    $root->right = $R->left;
    $R->left = $root;
    //這句必須啊！
    if ($R->parent == NULL) {
      $this->root = $R;
    }
  }
  /**
   * 查找樹中的最小關鍵字
   * @param $root 根節點
   * @return 最小關鍵字對應的節點
   */
  function search_min($root)
  {
    $current = $root;
    while ($current->left != NULL) {
      $current = $current->left;
    }
    return $current;
  }
  /**
   * 查找樹中的最大關鍵字
   * @param $root 根節點
   * @return 最大關鍵字對應的節點
   */
  function search_max($root)
  {
    $current = $root;
    while ($current->right != NULL) {
      $current = $current->right;
    }
    return $current;
  }
  /**
   * 查找某個$key在中序遍歷時的直接前驅節點
   * @param $x 待查找前驅節點的節點引用
   * @return 前驅節點引用
   */
  function predecessor($x)
  {
    //左子節點存在，直接返回左子節點的最右子節點
    if ($x->left != NULL) {
      return $this->search_max($x->left);
    }
    //否則查找其父節點，直到當前結點位于父節點的右邊
    $p = $x->parent;
    //如果x是p的左孩子，說明p是x的后繼，我們需要找的是p是x的前驅
    while ($p != NULL && $x == $p->left) {
      $x = $p;
      $p = $p->parent;
    }
    return $p;
  }
  /**
   * 查找某個$key在中序遍歷時的直接后繼節點
   * @param $x 待查找后繼節點的節點引用
   * @return 后繼節點引用
   */
  function successor($x)
  {
    if ($x->left != NULL) {
      return $this->search_min($x->right);
    }
    $p = $x->parent;
    while ($p != NULL && $x == $p->right) {
      $x = $p;
      $p = $p->parent;
    }
    return $p;
  }
  /**
   * 將$key插入樹中
   * @param $key 待插入樹的數字
   * @return null
   */
  public function Insert($key)
  {
    if (!is_null($this->search($key))) {
      throw new Exception('結點' . $key . '已存在，不可插入！');
    }
    $root = $this->root;
    $inode = new Node($key);
    $current = $root;
    $prenode = NULL;
    //為$inode找到合適的插入位置
    while ($current != NULL) {
      $prenode = $current;
      if ($current->key > $inode->key) {
        $current = $current->left;
      } else {
        $current = $current->right;
      }
    }
    $inode->parent = $prenode;
    //如果$prenode == NULL， 則證明樹是空樹
    if ($prenode == NULL) {
      $this->root = $inode;
    } else {
      if ($inode->key < $prenode->key) {
        $prenode->left = $inode;
      } else {
        $prenode->right = $inode;
      }
    }
    //將它重新修正為一顆紅黑樹
    $this->InsertFixUp($inode);
  }
  /**
   * 對插入節點的位置及往上的位置進行顏色調整
   * @param $inode 插入的節點
   * @return null
   */
  private function InsertFixUp($inode)
  {
    //情況一：需要調整條件，父節點存在且父節點的顏色是紅色
    while (($parent = $inode->parent) != NULL && $parent->IsRed == TRUE) {
      //祖父結點：
      $gparent = $parent->parent;
      //如果父節點是祖父結點的左子結點，下面的else與此相反
      if ($parent == $gparent->left) {
        //叔叔結點
        $uncle = $gparent->right;
        //case1:叔叔結點也是紅色
        if ($uncle != NULL && $uncle->IsRed == TRUE) {
          //將父節點和叔叔結點都涂黑，將祖父結點涂紅
          $parent->IsRed = FALSE;
          $uncle->IsRed = FALSE;
          $gparent->IsRed = TRUE;
          //將新節點指向祖父節點（現在祖父結點變紅，可以看作新節點存在）
          $inode = $gparent;
          //繼續while循環，重新判斷
          continue;  //經過這一步之后，組父節點作為新節點存在（跳到case2）
        }
        //case2:叔叔結點是黑色，且當前結點是右子節點
        if ($inode == $parent->right) {
          //以父節點作為旋轉結點做左旋轉處理
          $this->L_Rotate($parent);
          //在樹中實際上已經轉換，但是這里的變量的指向還沒交換，
          //將父節點和字節調換一下，為下面右旋做準備
          $temp = $parent;
          $parent = $inode;
          $inode = $temp;
        }
        //case3:叔叔結點是黑色，而且當前結點是父節點的左子節點
        $parent->IsRed = FALSE;
        $gparent->IsRed = TRUE;
        $this->R_Rotate($gparent);
      } //如果父節點是祖父結點的右子結點，與上面完全相反
      else {
        //叔叔結點
        $uncle = $gparent->left;
        //case1:叔叔結點也是紅色
        if ($uncle != NULL && $uncle->IsRed == TRUE) {
          //將父節點和叔叔結點都涂黑，將祖父結點涂紅
          $parent->IsRed = FALSE;
          $uncle->IsRed = FALSE;
          $gparent->IsRed = TRUE;
          //將新節點指向祖父節點（現在祖父結點變紅，可以看作新節點存在）
          $inode = $gparent;
          //繼續while循環，重新判斷
          continue;  //經過這一步之后，組父節點作為新節點存在（跳到case2）
        }
        //case2:叔叔結點是黑色，且當前結點是左子節點
        if ($inode == $parent->left) {
          //以父節點作為旋轉結點做右旋轉處理
          $this->R_Rotate($parent);
          //在樹中實際上已經轉換，但是這里的變量的指向還沒交換，
          //將父節點和字節調換一下，為下面右旋做準備
          $temp = $parent;
          $parent = $inode;
          $inode = $temp;
        }
        //case3:叔叔結點是黑色，而且當前結點是父節點的右子節點
        $parent->IsRed = FALSE;
        $gparent->IsRed = TRUE;
        $this->L_Rotate($gparent);
      }
    }
    //情況二：原樹是根節點（父節點為空），則只需將根節點涂黑
    if ($inode == $this->root) {
      $this->root->IsRed = FALSE;
      return;
    }
    //情況三：插入節點的父節點是黑色，則什么也不用做
    if ($inode->parent != NULL && $inode->parent->IsRed == FALSE) {
      return;
    }
  }
  /**
   * （對外）刪除指定節點
   * @param $key 刪除節點的key值
   * @return null
   */
  function Delete($key)
  {
    if (is_null($this->search($key))) {
      throw new Exception('結點' . $key . "不存在，刪除失敗！");
    }
    $dnode = $this->search($key);
    if ($dnode->left == NULL || $dnode->right == NULL) { #如果待刪除結點無子節點或只有一個子節點，則c = dnode
      $c = $dnode;
    } else { #如果待刪除結點有兩個子節點，c置為dnode的直接后繼，以待最后將待刪除結點的值換為其后繼的值
      $c = $this->successor($dnode);
    }
    //為了后面顏色處理做準備
    $parent = $c->parent;
    //無論前面情況如何，到最后c只剩下一邊子結點
    if ($c->left != NULL) {  //這里不會出現，除非選擇的是刪除結點的前驅
      $s = $c->left;
    } else {
      $s = $c->right;
    }
    if ($s != NULL) { #將c的子節點的父母結點置為c的父母結點，此處c只可能有1個子節點，因為如果c有兩個子節點，則c不可能是dnode的直接后繼
      $s->parent = $c->parent;
    }
    if ($c->parent == NULL) { #如果c的父母為空，說明c=dnode是根節點，刪除根節點后直接將根節點置為根節點的子節點，此處dnode是根節點，且擁有兩個子節點，則c是dnode的后繼結點，c的父母就不會為空，就不會進入這個if
      $this->root = $s;
    } else if ($c == $c->parent->left) { #如果c是其父節點的左右子節點，則將c父母的左右子節點置為c的左右子節點
      $c->parent->left = $s;
    } else {
      $c->parent->right = $s;
    }
    $dnode->key = $c->key;
    $node = $s;
    //c的結點顏色是黑色，那么會影響路徑上的黑色結點的數量，必須進行調整
    if ($c->IsRed == FALSE) {
      $this->DeleteFixUp($node,$parent);
    }
  }
  /**
   * 刪除節點后對接點周圍的其他節點進行調整
   * @param $key 刪除節點的子節點和父節點
   * @return null
   */
  private function DeleteFixUp($node,$parent)
  {
    //如果待刪結點的子節點為紅色，直接將子節點涂黑
    if ($node != NULL && $node->IsRed == TRUE) {
      $node->IsRed = FALSE;
      return;
    }
    //如果是根節點，那就直接將根節點置為黑色即可
    while (($node == NULL || $node->IsRed == FALSE) && ($node != $this->root)) {
      //node是父節點的左子節點，下面else與這里相反
      if ($node == $parent->left) {
        $brother = $parent->right;
        //case1:兄弟結點顏色是紅色（父節點和兄弟孩子結點都是黑色）
        //將父節點涂紅，將兄弟結點涂黑，然后對父節點進行左旋處理（經過這一步，情況轉換為兄弟結點顏色為黑色的情況）
        if ($brother->IsRed == TRUE) {
          $brother->IsRed = FALSE;
          $parent->IsRed = TRUE;
          $this->L_Rotate($parent);
          //將情況轉化為其他的情況
          $brother = $parent->right; //在左旋處理后，$parent->right指向的是原來兄弟結點的左子節點
        }
        //以下是兄弟結點為黑色的情況
        //case2:兄弟結點是黑色，且兄弟結點的兩個子節點都是黑色
        //將兄弟結點涂紅，將當前結點指向其父節點，將其父節點指向當前結點的祖父結點。
        if (($brother->left == NULL || $brother->left->IsRed == FALSE) && ($brother->right == NULL || $brother->right->IsRed == FALSE)) {
          $brother->IsRed = TRUE;
          $node = $parent;
          $parent = $node->parent;
        } else {
          //case3:兄弟結點是黑色，兄弟結點的左子節點是紅色，右子節點為黑色
          //將兄弟結點涂紅，將兄弟節點的左子節點涂黑，然后對兄弟結點做右旋處理（經過這一步，情況轉換為兄弟結點顏色為黑色，右子節點為紅色的情況）
          if ($brother->right == NULL || $brother->right->IsRed == FALSE) {
            $brother->IsRed = TRUE;
            $brother->left->IsRed = FALSE;
            $this->R_Rotate($brother);
            //將情況轉換為其他情況
            $brother = $parent->right;
          }
          //case4:兄弟結點是黑色，且兄弟結點的右子節點為紅色，左子節點為任意顏色
          //將兄弟節點涂成父節點的顏色，再把父節點涂黑，將兄弟結點的右子節點涂黑，然后對父節點做左旋處理
          $brother->IsRed = $parent->IsRed;
          $parent->IsRed = FALSE;
          $brother->right->IsRed = FALSE;
          $this->L_Rotate($parent);
          //到了第四種情況，已經是最基本的情況了，可以直接退出了
          $node = $this->root;
          break;
        }
      } //node是父節點的右子節點
      else {
        $brother = $parent->left;
        //case1:兄弟結點顏色是紅色（父節點和兄弟孩子結點都是黑色）
        //將父節點涂紅，將兄弟結點涂黑，然后對父節點進行右旋處理（經過這一步，情況轉換為兄弟結點顏色為黑色的情況）
        if ($brother->IsRed == TRUE) {
          $brother->IsRed = FALSE;
          $parent->IsRed = TRUE;
          $this->R_Rotate($parent);
          //將情況轉化為其他的情況
          $brother = $parent->left; //在右旋處理后，$parent->left指向的是原來兄弟結點的右子節點
        }
        //以下是兄弟結點為黑色的情況
        //case2:兄弟結點是黑色，且兄弟結點的兩個子節點都是黑色
        //將兄弟結點涂紅，將當前結點指向其父節點，將其父節點指向當前結點的祖父結點。
        if (($brother->left == NULL || $brother->left->IsRed == FALSE) && ($brother->right == NULL || $brother->right->IsRed == FALSE)) {
          $brother->IsRed = TRUE;
          $node = $parent;
          $parent = $node->parent;
        } else {
          //case3:兄弟結點是黑色，兄弟結點的右子節點是紅色，左子節點為黑色
          //將兄弟結點涂紅，將兄弟節點的左子節點涂黑，然后對兄弟結點做左旋處理（經過這一步，情況轉換為兄弟結點顏色為黑色，右子節點為紅色的情況）
          if ($brother->left == NULL || $brother->left->IsRed == FALSE) {
            $brother->IsRed = TRUE;
            $brother->right = FALSE;
            $this->L_Rotate($brother);
            //將情況轉換為其他情況
            $brother = $parent->left;
          }
          //case4:兄弟結點是黑色，且兄弟結點的左子節點為紅色，右子節點為任意顏色
          //將兄弟節點涂成父節點的顏色，再把父節點涂黑，將兄弟結點的右子節點涂黑，然后對父節點左左旋處理
          $brother->IsRed = $parent->IsRed;
          $parent->IsRed = FALSE;
          $brother->left->IsRed = FALSE;
          $this->R_Rotate($parent);
          $node = $this->root;
          break;
        }
      }
    }
    if ($node != NULL) {
      $this->root->IsRed = FALSE;
    }
  }
  /**
   * （對內）獲取樹的深度
   * @param $root 根節點
   * @return 樹的深度
   */
  private function getdepth($root)
  {
    if ($root == NULL) {
      return 0;
    }
    $dl = $this->getdepth($root->left);
    $dr = $this->getdepth($root->right);
    return ($dl > $dr ? $dl : $dr) + 1;
  }
  /**
   * （對外）獲取樹的深度
   * @param null
   * @return null
   */
  public function Depth()
  {
    return $this->getdepth($this->root);
  }
}
?>

php是什么語言

php，一個嵌套的縮寫名稱，是英文超級文本預處理語言（PHP:Hypertext Preprocessor）的縮寫。PHP 是一種 HTML 內嵌式的語言，PHP與微軟的ASP頗有幾分相似，都是一種在服務器端執行的嵌入HTML文檔的腳本語言，語言的風格有類似于C語言，現在被很多的網站編程人員廣泛的運用。

以上是“PHP如何實現繪制二叉樹圖形顯示功能”這篇文章的所有內容，感謝各位的閱讀！希望分享的內容對大家有幫助，更多相關知識，歡迎關注億速云行業資訊頻道！